
We can define these equations in Mathematica with the following commands: You've probably seen the parametric equations for the unit circle before:, where 0≤t≤2π. Using parametric equations can solve this problem. We've just pasted together two graphs to make it look like one circle.
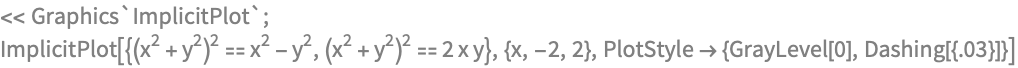
So we do in fact get to see the whole circle, but we haven't really graphed the circle. If we wish to see the whole circle, we can show the two graphs together: (The AspectRatio→Automatic option forces Mathematica to scale the graph so it looks like a piece of a circle try removing the option to see what happens.) We can plot these separately, but they each represent only half of the circle:

To use this command we need to solve our equation for y, and this is where the trouble starts we get two solutions, and. You know from Lab 1A that one way to graph a function y=f(x) is the Mathematica command Plot. For example,consider the unit circle + =1. Why do we care about parametric equations? There are many reasons, but one of the most important is that using parametric equations allows us to graph things that we otherwise wouldn't be able to graph. The middle picture is a closed curve the others are not. The following pictures are all examples of curves. In this case we call the curve a closed curve. Sometimes the curve will have two ends sometimes the two ends of the curve will actually be the same point - i.e.the two ends of the string are taped together. If you like, you could think of a curve as a piece of string that's sitting in two- or three-dimensional space.Įxamples are a segment of a straight line, a circle, or a section of any graph of a function. The term "curve" has not been defined yet, but roughly speaking,a curve (or an "arc") is a curvy line in space.

This week we will consider parametric equations of curve. These skills will be very important later in this class,which is why we're spending so much time on them now. Instead, it's about visualizing and describing different curves and surfaces. Questions to: than a short bit about derivatives at the end of this notebook, Lab 2 is almost entirely devoid of calculus.


 0 kommentar(er)
0 kommentar(er)
